The above property is exclusive to right triangles, and there are many other characterizations of right triangles, as seen in this list.
Today’s post will push at least two more entries into the list, in addition to that of July 14 ![]() .
.
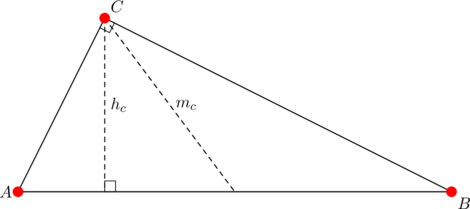
Consider a right triangle ![]() in which
in which ![]() as shown below:
as shown below:

The length of the median to the hypotenuse is half the length of the hypotenuse: ![]() . Thus, the area is:
. Thus, the area is:
![]()
Suppose that the area of ![]() is equal to the product of the altitude
is equal to the product of the altitude ![]() and median
and median ![]() from the same vertex
from the same vertex ![]() . Let
. Let ![]() be the side-lengths and let
be the side-lengths and let ![]() be the circumradius. Then
be the circumradius. Then
![]()
The area can be given as ![]() , and
, and ![]() itself can be written as
itself can be written as ![]() , by the extended law of sines. So:
, by the extended law of sines. So:
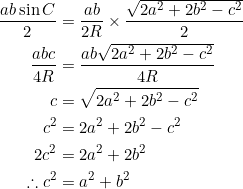
Let ![]() be the circumradius of the parent triangle
be the circumradius of the parent triangle ![]() . If the circle with diameter
. If the circle with diameter ![]() coincides with the nine-point circle, then we must have
coincides with the nine-point circle, then we must have ![]() , since the radius of the nine-point circle is
, since the radius of the nine-point circle is ![]() .
.
![]()
the latter equation is one of the characterizations of right triangles given here.
In the next example we have a weaker requirement — we just ask that the nine-point circle pass through ![]() or
or ![]() .
.
Suppose that the nine-point circle passes through ![]() . Let
. Let ![]() be the nine-point center. Then
be the nine-point center. Then ![]() is a radius of the nine-point circle, namely
is a radius of the nine-point circle, namely ![]() . But then
. But then ![]() .
.
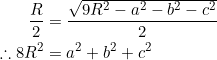
Similarly, if the nine-point circle of a triangle passes through the orthocenter of the triangle, then the parent triangle must be a right triangle.
of
The harmonic mean of ![]() and
and ![]() is
is ![]() . The square of the length of the bisector of
. The square of the length of the bisector of ![]() is given by
is given by ![]() . We get
. We get
![Rendered by QuickLaTeX.com \[\left(1-\left(\frac{c}{a+b}\right)^2\right)=\frac{1}{2}\left(\frac{2ab}{a+b}\right)^2\]](https://blog.fridaymath.com/wp-content/ql-cache/quicklatex.com-db0b4b3cd169f2e9b5966ffaece45c26_l3.png)
and then finally ![]() after simplifications.
after simplifications.
Takeaway
In ![]() , let
, let ![]() be the side-lengths,
be the side-lengths, ![]() the median from vertex
the median from vertex ![]() ,
, ![]() the altitude from vertex
the altitude from vertex ![]() ,
, ![]() the orthocenter, and
the orthocenter, and ![]() the circumcenter. Then the following statements are equivalent:
the circumcenter. Then the following statements are equivalent:
- the area of
 equals
equals 
 is a right triangle with
is a right triangle with 
- the nine-point circle of
 passes through
passes through 
- the nine-point circle of
 passes through
passes through 
- the circle with diameter
 coincides with the nine-point circle of
coincides with the nine-point circle of 
 is the geometric mean of the two equal segments it creates on the opposite side
is the geometric mean of the two equal segments it creates on the opposite side 
- the square of the length of the bisector of angle
 is half the square of the harmonic mean of
is half the square of the harmonic mean of  and
and  .
.
In the case of statement 6, one can replace the geometric mean with arithmetic mean or harmonic mean. Why?
Task
- (Early fifties) In a non-right triangle
 , let
, let  be the side-lengths,
be the side-lengths,  the altitudes,
the altitudes,  the feet of the altitudes from the respective vertices,
the feet of the altitudes from the respective vertices,  the circumradius,
the circumradius,  the circumcenter,
the circumcenter,  the nine-point center,
the nine-point center,  the orthocenter,
the orthocenter,  the midpoint of side
the midpoint of side  , and
, and  the reflection of
the reflection of  over side
over side  . PROVE that the following fifty-four statements are equivalent:
. PROVE that the following fifty-four statements are equivalent:






























 is congruent to
is congruent to 
 is isosceles with
is isosceles with 
 is isosceles with
is isosceles with 
 is right angled at
is right angled at 
 is the circumcenter of
is the circumcenter of 
 is right-angled at
is right-angled at 
 is right-angled at
is right-angled at 
- quadrilateral
 is a rectangle
is a rectangle - the points
 are concyclic with
are concyclic with  as diameter
as diameter - the reflection of
 over
over  lies internally on
lies internally on 
- the reflection of
 over
over  lies externally on
lies externally on 
- radius
 is parallel to side
is parallel to side 
 is the reflection of
is the reflection of  over side
over side 
- the nine-point center lies on

- the orthic triangle is isosceles with

- the geometric mean theorem holds
- the bisector of
 has length
has length  , where
, where 
- the orthocenter is a reflection of vertex
 over side
over side 
- segment
 is tangent to the circumcircle at point
is tangent to the circumcircle at point 
- median
 has the same length as the segment
has the same length as the segment 
- the bisector
 of
of  is tangent to the nine-point circle at
is tangent to the nine-point circle at 
 is a convex kite with diagonals
is a convex kite with diagonals  and
and 
- altitude
 is tangent to the nine-point circle at
is tangent to the nine-point circle at 
- segment
 is tangent to the nine-point circle at
is tangent to the nine-point circle at  .
.
( short of the target.)
short of the target.)
- (Extra feature) If
 satisfies equation (??), PROVE that its nine-point center
satisfies equation (??), PROVE that its nine-point center  divides
divides  in the ratio
in the ratio  .
.