The Euler line as a diameter II – High School Geometry

Let $O$

and $H$

be the circumcenter and orthocenter of $\triangle ABC$

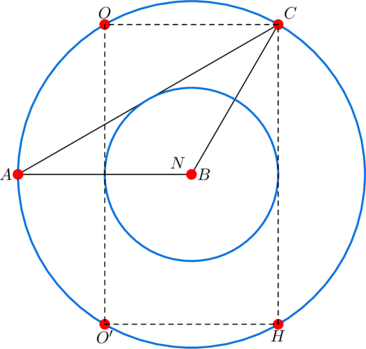
Last time we saw that the circle with diameter $OH$ passes through vertex $C$ if and only if the equation below holds:

(1) $\begin{equation*} (a^2-b^2)^2=(ac)^2+(cb)^2 \end{equation*}$

Let this circle also pass through vertex $A$ . Then the nine-point center has to be $B$ .

Updated equivalence

We now add the following four equivalent statements to what we had at the end of our post on August 14:

a circle with diameter $OH$ passes through $A$ and $C$
the nine-point center coincides with vertex $B$
the reflection of $O$ over $AC$ is $B$
the reflection of $H$ over $BC$ is $A$

Only triangle $ABC$ having $a:b:c=1:\sqrt{3}:1$ satisfies these.

In $\triangle ABC$

, if the circle with diameter $OH$

passes through $A$

and $C$

, PROVE that the nine-point center is $B$

Since this circle passes through vertex $C$ , our previous post shows that equation (1) is satisfied:

$(a^2-b^2)^2=(ac)^2+(cb)^2$

Further, with $OH$ as diameter, the two triangles $CHO$ and $AHO$ are right triangles with $\angle HAO=90^{\circ}$ and $\angle HCO=90^{\circ}$ .

$\begin{equation*} \begin{split} OH^2&=OA^2+AH^2\\ 9R^2-a^2-b^2-c^2&=R^2+(4R^2-a^2)\\ \therefore b^2+c^2&=4R^2\\ \vdots&\vdots\\ OH^2&=OC^2+CH^2\\ 9R^2-a^2-b^2-c^2&=R^2+(4R^2-c^2)\\ \therefore a^2+b^2&=4R^2\\ \vdots&\vdots\\ \therefore a&=c \end{split} \end{equation*}$

By a result in this post, we conclude that the nine-point center is $B$ .

If the nine-point center of $\triangle ABC$

coincides with vertex $B$

, PROVE that the reflection of the circumcenter $O$

over side $AC$

is $B$

Easy.

If the reflection of the circumcenter $O$

over $AC$

is $B$

, PROVE that the reflection of the orthocenter $H$

over $BC$

is $A$

Easy.

If the reflection of $H$

over $BC$

is $A$

, PROVE that the circle with diameter $OH$

passes through $A$

and $C$

Easy.

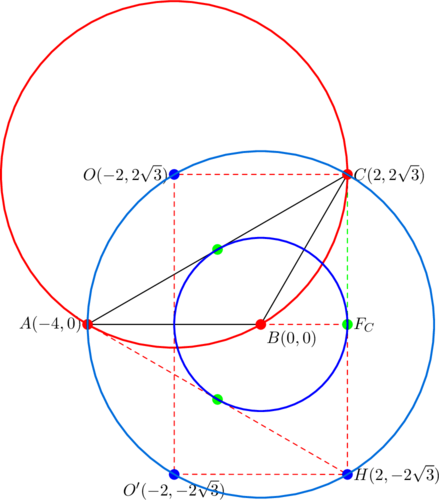
Usual example

Consider $\triangle ABC$

with vertices at $A(-4,0)$

, $B(0,0)$

, and $C(2,2\sqrt{3})$

. Its nine-point center is $B$

, and so it satisfies all the equivalent statements listed above.

The diagram below shows the circumcircle, the nine-point circle, and the circle with diameter $OH$ .

Or this one:

Takeaway

Consider $\triangle ABC$ with side-lengths $a,b,c$ , circumradius $R$ , circumcenter $O$ , orthocenter $H$ , and nine-point center $N$ . If equation (1) is satisfied, then the following statements are equivalent:

a circle with diameter $OH$ passes through $A$ and $C$
$\triangle OAC$ and $\triangle OBC$ are both equilateral
$HA$ is tangent to the circumcircle at $A$
the orthic triangle is equilateral
the reflection of $O$ over $AC$ is $B$
the reflection of $H$ over $BC$ is $A$
$\triangle ACH$ is equilateral
$N$ coincides with $B$
$a:b:c=1:\sqrt{3}:1$
$a=c=R$

These are some of the many equivalent descriptions of the isosceles triangle $ABC$ in which $\angle A=\angle C=30^{\circ}$ .

Task

(Early fifties) In a non-right triangle , let be the side-lengths, the altitudes, the feet of the altitudes from the respective vertices, the circumradius, the circumcenter, the nine-point center, the orthocenter, the midpoint of side , and the reflection of over side . PROVE that the following fifty-two statements are equivalent:
1. $AH=b$
2. $BH=a$
3. $CH=2h_C$
4. $h_A=AF_B$
5. $h_B=BF_A$
6. $AF_C=\frac{b^2}{2R}$
7. $BF_C=\frac{a^2}{2R}$
8. $\frac{a}{c} =\frac{h_C}{AF_B}$
9. $\frac{b}{c}=\frac{h_C}{BF_A}$
10. $\frac{a}{b}=\frac{BF_A}{AF_B}$
11. $R=\frac{|a^2-b^2|}{2c}$
12. $h_C=R\cos C$
13. $\cos A=\frac{b(b^2-a^2)}{c(a^2+b^2)}$
14. $\cos B=\frac{a(a^2-b^2)}{c(a^2+b^2)}$
15. $\cos C=\frac{2ab}{a^2+b^2}$
16. $\cos^2 A+\cos^2 B=1$
17. $\sin^2 A+\sin^2 B=1$
18. $a\cos A+b\cos B=0$
19. $2\cos A\cos B+\cos C=0$
20. $a\cos A-b\cos B=\sqrt{a^2+b^2}\cos C$
21. $OH^2=5R^2-c^2$
22. $h_A^2+h_B^2=AB^2$
23. $\frac{h_A}{a}+\frac{h_B}{b}=\frac{c}{h_C}$
24. $a^2+b^2=4R^2$
25. $A-B=\pm 90^{\circ}$
26. $(a^2-b^2)^2=(ac)^2+(cb)^2$
27. $AH^2+BH^2+CH^2=8R^2-c^2$
28. $a=2R\sin A,~b=2R\cos A,~c=2R\cos 2A$
29. $\triangle ABH$ is congruent to $\triangle ABC$
30. $\triangle CNO$ is isosceles with $CN=NO$
31. $\triangle CNH$ is isosceles with $CN=NH$
32. $\triangle CHO$ is right angled at $C$
33. $N$ is the circumcenter of $\triangle CHO$
34. $\triangle O'OC$ is right-angled at $O$
35. $\triangle O'HC$ is right-angled at $H$
36. quadrilateral $O'OHC$ is a rectangle
37. the points $O',O,C,H$ are concyclic with $OH$ as diameter
38. the reflection of $O$ over $AC$ lies internally on $AB$
39. the reflection of $O$ over $BC$ lies externally on $AB$
40. radius $OC$ is parallel to side $AB$
41. $F_A$ is the reflection of $F_B$ over side $AB$
42. the nine-point center lies on $AB$
43. the orthic triangle is isosceles with $F_AF_C=F_BF_C$
44. the geometric mean theorem holds
45. the bisector of $\angle C$ has length $l$ , where $l^2=\frac{2a^2b^2}{a^2+b^2}$
46. the orthocenter is a reflection of vertex $C$ over side $AB$
47. segment $HC$ is tangent to the circumcircle at point $C$
48. median $CM$ has the same length as the segment $HM$
49. the bisector $MO$ of $AB$ is tangent to the nine-point circle at $M$
50. $AF_ABF_B$ is a convex kite with diagonals $AB$ and $F_AF_B$
51. altitude $CF_C$ is tangent to the nine-point circle at $F_C$
52. segment $HF_C$ is tangent to the nine-point circle at $F_C$ .
  ( $23$ short of the target.)
(Extra feature) If $\triangle ABC$ satisfies equation (??), PROVE that its nine-point center $N$ divides $AB$ in the ratio $|3b^2-a^2|:|b^2-3a^2|$ .