Congruent triangles
Let’s prove one direction: ![]() (1). Consider
(1). Consider ![]() shown below:
shown below:
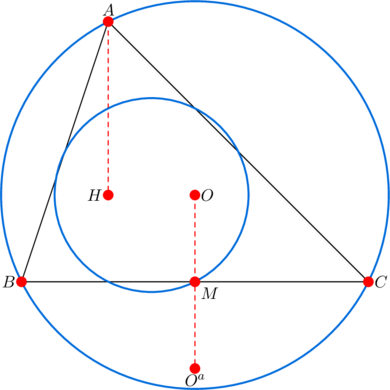
We showed previously that ![]() in any triangle with orthocenter
in any triangle with orthocenter ![]() . Thus
. Thus ![]() . By one of the equivalent statements here, we conclude that equation (1) holds.
. By one of the equivalent statements here, we conclude that equation (1) holds.
Similar to example 1 above. If ![]() is the reflection of the circumcenter over side
is the reflection of the circumcenter over side ![]() , then we always have
, then we always have ![]() in any triangle. This would then lead to
in any triangle. This would then lead to ![]() , which by the equivalent statements here implies equation (1).
, which by the equivalent statements here implies equation (1).
This follows from the two preceding examples, together with the fact that the segment ![]() has length
has length ![]() . (Recall that
. (Recall that ![]() is congruent to the parent
is congruent to the parent ![]() in such a way that
in such a way that ![]() ,
, ![]() , and
, and ![]() .)
.)
Cyclic trapezium
Note that under the assumption of equation (1), we have that the reflection ![]() lies on side
lies on side ![]() . Let
. Let ![]() be the circumradius of the parent triangle
be the circumradius of the parent triangle ![]() . Then
. Then ![]() and also
and also ![]() . Since radius
. Since radius ![]() is parallel to side
is parallel to side ![]() (see here), we get an isosceles trapezium
(see here), we get an isosceles trapezium ![]() .
.
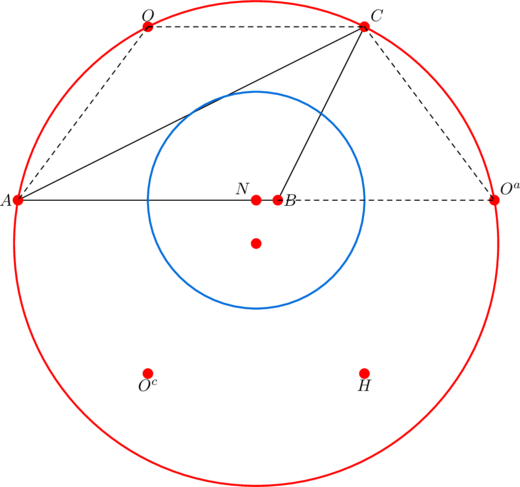
Similar to example 4 above.
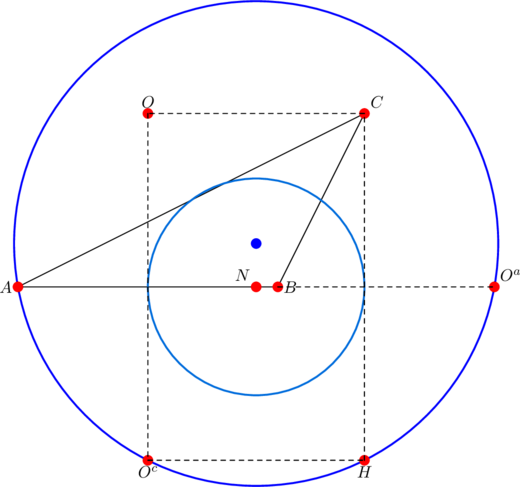
Takeaway
In ![]() , let
, let ![]() be the side-lengths,
be the side-lengths, ![]() the circumcenter,
the circumcenter, ![]() the reflection of
the reflection of ![]() over side
over side ![]() ,
, ![]() the reflection of
the reflection of ![]() over side
over side ![]() , and
, and ![]() the reflection of
the reflection of ![]() over side
over side ![]() . Then the following statements are equivalent:
. Then the following statements are equivalent:
As usual, we have more of these in the exercises.
Task
- (Mid fifties) In a non-right triangle
 , let
, let  be the side-lengths,
be the side-lengths,  the altitudes,
the altitudes,  the feet of the altitudes from the respective vertices,
the feet of the altitudes from the respective vertices,  the circumradius,
the circumradius,  the circumcenter,
the circumcenter,  the nine-point center,
the nine-point center,  the orthocenter,
the orthocenter,  the midpoint of side
the midpoint of side  ,
,  the reflection of
the reflection of  over side
over side  ,
,  the reflection of
the reflection of  over side
over side  , and
, and  the reflection of
the reflection of  over side
over side  . PROVE that the following fifty-six statements are equivalent:
. PROVE that the following fifty-six statements are equivalent:
































 is congruent to
is congruent to 
 is isosceles with
is isosceles with 
 is isosceles with
is isosceles with 
 is right angled at
is right angled at 
 is the circumcenter of
is the circumcenter of 
 is right-angled at
is right-angled at 
 is right-angled at
is right-angled at 
- quadrilateral
 is a rectangle
is a rectangle - the points
 are concyclic with
are concyclic with  as diameter
as diameter - the reflection
 of
of  over
over  lies internally on
lies internally on 
- the reflection
 of
of  over
over  lies externally on
lies externally on 
- radius
 is parallel to side
is parallel to side 
 is the reflection of
is the reflection of  over side
over side 
- the nine-point center lies on

- the orthic triangle is isosceles with

- the geometric mean theorem holds
- the bisector of
 has length
has length  , where
, where 
- the orthocenter is a reflection of vertex
 over side
over side 
- segment
 is tangent to the circumcircle at point
is tangent to the circumcircle at point 
- median
 has the same length as the segment
has the same length as the segment 
- the bisector
 of
of  is tangent to the nine-point circle at
is tangent to the nine-point circle at 
 is a convex kite with diagonals
is a convex kite with diagonals  and
and 
- altitude
 is tangent to the nine-point circle at
is tangent to the nine-point circle at 
- segment
 is tangent to the nine-point circle at
is tangent to the nine-point circle at  .
.
( short of the target.)
short of the target.)
- (Extra feature) If
 satisfies equation (??), PROVE that its nine-point center
satisfies equation (??), PROVE that its nine-point center  divides
divides  in the ratio
in the ratio  .
.