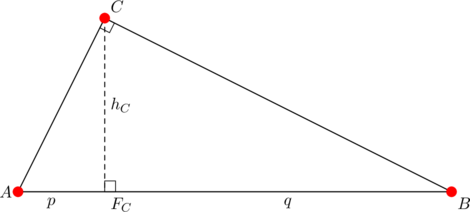
the geometric mean theorem states that
(1) ![]()
As shown here, equation (1) is equivalent to the Pythagorean identity:
(2) ![]()
However, the equivalence holds because the altitude is internal. In the case of an external altitude, we present an analogous geometric mean theorem:

(3) ![]()
Here, equation (3) is equivalent to:
(4) ![]()

We have
![]()
External altitudes
In examples 2 and 3 below, we prove the equivalence between equations (3) and (4).

Apply the Pythagorean theorem to triangles ![]() and
and ![]() :
:
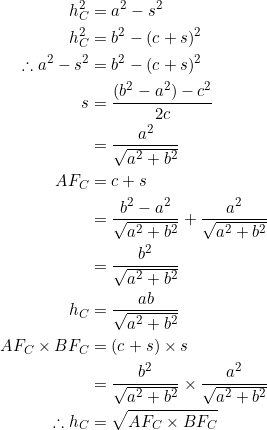

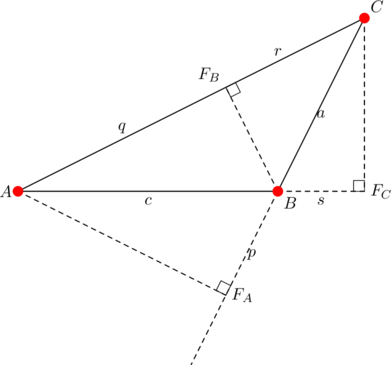
Assume that in the diagram above one has ![]() . As before,
. As before, ![]() , giving
, giving
![]()
![Rendered by QuickLaTeX.com \begin{equation*} \begin{split} h_C^2&=AF_C\times BF_C\\ \therefore a^2-\left(\frac{b^2-a^2-c^2}{2c}\right)^2&=\scriptstyle\left(\frac{b^2-a^2+c^2}{2c}\right)\left(\frac{b^2-a^2-c^2}{2c}\right)\\ 4a^2c^2-\left[(b^2-a^2)^2+c^4-2c^2(b^2-a^2)\right]&=(b^2-a^2)^2-c^4\\ \therefore c^2(a^2+b^2)&=(b^2-a^2)^2\\ \end{split} \end{equation*}](https://blog.fridaymath.com/wp-content/ql-cache/quicklatex.com-eedb72735f717f000666f41f5a429db7_l3.png)
Extra attributes
When a triangle satisfies equation (4), it is peculiar in its own right, but also shares some things in common with the right triangle.
Note that (by definition) ![]() and
and ![]() , where
, where ![]() and
and ![]() are the lengths of the altitudes from
are the lengths of the altitudes from ![]() and
and ![]() . From the above diagram:
. From the above diagram:
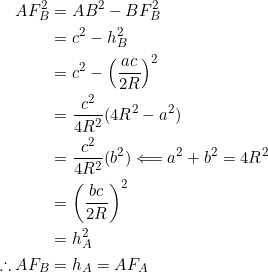
Similarly, ![]() .
.

From the previous example we had ![]() and
and ![]() , so:
, so:
![]()
From the diagram
![Rendered by QuickLaTeX.com \[s=\sqrt{a^2-h_C^2}=\sqrt{a^2-\left(\frac{ab}{2R}\right)^2}=\frac{a^2}{2R}\implies cs=\frac{a^2c}{2R}.\]](https://blog.fridaymath.com/wp-content/ql-cache/quicklatex.com-966fecb9c492fcc3c8f9a898e2ce45e6_l3.png)

Note that ![]() (example 4) and
(example 4) and ![]() , where
, where ![]() is the circumradius.
is the circumradius.
![]()
And
![]()
(Along the line we used equation (4) and its equivalent representation ![]() .)
.)
Conclusion: ![]() or
or ![]() .
.
The relation ![]() also holds for right triangles. Using equation (4) and the cosine formula, we obtain
also holds for right triangles. Using equation (4) and the cosine formula, we obtain
![]()
This is probably the easiest way to see the relationship between right triangles and triangles satisfying equation (4).
From example 7 above we had ![]() . But then
. But then ![]() also, giving
also, giving ![]() .
.
![]()
Our triangle is obtuse so we choose ![]() .
.
Using ![]() , the cosine formula, and skipping a couple of steps, we have:
, the cosine formula, and skipping a couple of steps, we have:
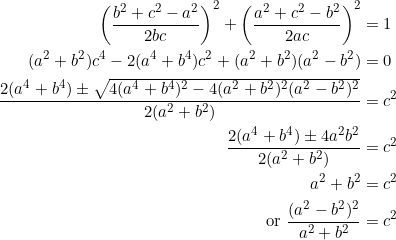
The second possibility ![]() is just equation (4) re-arranged.
is just equation (4) re-arranged.
Essential awareness
The preceding examples show that our newest arrival — any ![]() satisfying equation (4) –“rivals” the right triangle in many aspects. More on that later. For now, any triangle whose side-slopes form a geometric progression is also comparable to a right triangle. There, a modified geometric mean theorem holds (see example 10).
satisfying equation (4) –“rivals” the right triangle in many aspects. More on that later. For now, any triangle whose side-slopes form a geometric progression is also comparable to a right triangle. There, a modified geometric mean theorem holds (see example 10).
(5) ![]()
where ![]() and
and ![]() .
.
Earlier aim
Our original plan was to focus on equation (5) throughout today, but we were redirected along the way. Kindly bear with us for not completely fulfilling the promise made in example 8 of our post on December 28, 2020.
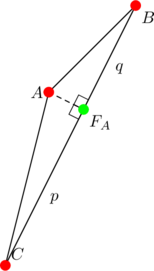
The foot of the altitude from ![]() is
is ![]() , as shown above. By the distance formula:
, as shown above. By the distance formula:
![]()
The slopes of the sides of ![]() form a geometric progression with
form a geometric progression with ![]() and
and ![]() , so we can now
, so we can now ![]() al
al![]() ulate
ulate ![]() :
:
![]()
Finally we compare both ![]() and
and ![]() :
:
![]()
Equal. Equation (5) is satisfied.
Takeaway
For any obtuse triangle ![]() , the following statements are equivalent:
, the following statements are equivalent:

- the orthic triangle is isosceles
- the geometric mean theorem holds.
We have a longer chain of equivalence in the exercises below.
Tasks
- (Long chain) For any obtuse triangle
 , PROVE that each of the following statements implies the others:
, PROVE that each of the following statements implies the others:












 or
or 
- the orthic triangle is isosceles
- the geometric mean theorem holds.
(Some of the statements above are satisfied in a right triangle, but the entire chain of statements are no longer equivalent in that case.) For something different, some of the above statements are clearly redundant; notwithstanding, just admire the sheer quantity. The aim is to increase the numbers until as many statements as there are in the Invertible Matrix Theorem are reached (and “breached”).
- (Linear combination) Suppose that
 satisfies equation (4).
satisfies equation (4).
- PROVE that
 .
. - Write
 in the form
in the form  .
.
- PROVE that
- Consider
 with vertices at
with vertices at  ,
,  ,
,  . Denote its orthocenter and circumcenter by
. Denote its orthocenter and circumcenter by  and
and  as usual.
as usual.
- Verify that
 . Hence, deduce that
. Hence, deduce that  (in other words, equation (4) is satisfied)
(in other words, equation (4) is satisfied) - PROVE that
 . Hence, deduce that radius
. Hence, deduce that radius  is parallel to side
is parallel to side 
- PROVE that
 . Deduce that the orthocenter is the reflection of vertex
. Deduce that the orthocenter is the reflection of vertex  over side
over side  .
.
- Verify that
- PROVE that the two statements below are equivalent for a triangle
 :
:
- it is a right triangle
- its area is
 , the product of the altitude and median from the same vertex.
, the product of the altitude and median from the same vertex.
- Consider
 with side-lengths
with side-lengths  and altitudes
and altitudes  .
.